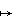Isometries of n-dimensional space

We are now in a position to calculate the group of Euclidean geometry.

Definition

If a ∈ Rⁿ then the map x 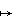x + a is called a translation and is denoted by T_a.

Note that translations and orthogonal (linear) transformations are both isometries.

In fact we have the following important result.

Theorem
The group I(Rⁿ) of all isometries of Rⁿ consists of composites T_a L where T_a is a translation and L is an orthogonal map.

Proof
If f : Rⁿ→ Rⁿ is an isometry then let f(0) = a.
Then T_-a f maps 0 to 0. Our result will then follow from the following:

Lemma
An isometry L which fixes 0 is a linear map.

Proof of lemma
Since a straight line can be defined in terms of minimising distance, any isometry maps straight lines to straight lines.
Also parallel lines are mapped to parallel lines and hence parallelograms are mapped to parallelograms.
Hence L(a + b) = L(a) + L(b) since addition is defined using the parallelogram law.
To show that L(λa) = λL(a) observe that this is true for λ = 1 and hence (by adding) for any λ ∈ Z and hence for λ ∈ Q. Note that any isometry is clearly continuous and so continuity then allows us to extend it to the whole of R.
This completes the proof of the lemma and (since any length preserving linear map is orthogonal) of the theorem.


Note that the set of all orthogonal transformations forms a subgroup of I(Rⁿ). We also have:

Theorem
The set of all translations forms a normal subgroup of I(Rⁿ) which is isomorphic to the group Rⁿ under addition.

Proof
Clearly the set of translations forms a subgroup isomorphic to Rⁿ. To show it is normal, conjugate a translation T_b by an element f = T_a L of I(Rⁿ).
f^-1 T_b f(x) = L^-1 T_-a T_b T_a L(x) = L^-1(b + L(x)) = L^-1(b) + x which is translation by L^-1(b) and so is in the subgroup.


Remark

In fact the quotient group is isomorphic to the group O(n). However, in general the subgroup O(n) of I(Rⁿ) is not a normal subgroup and so the group of isometries is not the direct product of these two subgroups.
In fact "as a space" I(Rⁿ) is Rⁿ × O(n) but the group multiplication is not componentwise.