Solution 9

1. 
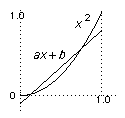
   [d₂(x , ax + b)]²= (x² - (ax + b)²dx = (x⁴ - 2x²(ax + b) + (ax + b)²) dx = ¹/₅ - ²/₄a -²/₃ b + ¹/₃ a² + ab + b². The minimum value of this is where ^d/_da = 0 and ^d/_db = 0. That is where -¹/₂ + ²/₃a + b = 0 and -²/₃ + a + 2b = 0 ⇒ 4a + 6b = 3, 3a + 6b = 2 ⇒ a = 1, b = -1/6. So the best-fit linear approximation to x² is x - ¹/₆.

2. Let _n be the partial sum of 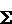f_i, assume that m > n and look at d_∞(_m- _n) = max { |f_n+1(x) + ... + f_m(x)| for x ∈ [a, b]} ≤ max {|f_n+1(x)| + ... + |f_m(x)| } ≤ M_n+1+ ... + M_m.
   Since the series 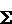M_i converges, its partial sums form a Cauchy sequence and so M_n+1+ ... + M_m is small for large m, n. Thus (_n) is a Cauchy sequence in C[a, b] and since this is a complete space under d_∞ the sequence is convergent to its pointwise limit and this limit is continuous.
   
   Since |sin²(x)| ≤ 1, we may take M_n= 1/n² and so by the Weierstrass M-test, the series 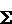sin²(x)/n² is uniformly convergent to a continuous function.
   Deciding exactly where this function is differentiable is much harder.
   
   Similarly, since |cos(aⁿx)| ≤ 1, if 0 < b < 1 we may take M_n= 1/bⁿ and use the fact that 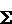M_n is convergent to deduce that Weierstrass's function is continuous.
   Proving that this function is not differentiable anywhere is the hard bit!
   
   

3. It is clear that d(m, n) ≥ 0, that d(m, n) = 0 ⇔ m = n and that d(m, n) = d(n, m), so we need only verify the triangle inequality.
   So suppose that 2^r divides m - n and that 2^s divides n - q. Then m - q = (m - n) + (n - q) and so if t = min{r, s} we have 2^t divides n - q. But 1/2^t≤ 1/2^r+ 1/2^s since t is either r or s. Hence the triangle inequality holds.
   
   If a_n= 1/2^n-1 then d(a_n, 0) = 1/2^n-1 and so (a_n)→ 0 in this metric.
   
   One can define similar metrics for any odd prime in a similar way. This gives rise to some interesting algebraic objects called the p-adic integers which arise in a wide variety of applications.