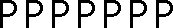 ....
....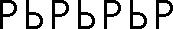 ....
....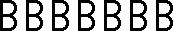 ....
.... ....
.... ....
.... ....
....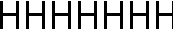 ....
....| Previous page (Symmetry groups of plane figures) | Contents | Next page (Symmetry groups of Platonic solids) |
We now look at some infinite subgroups of I(R2).
We will look at subgroups which map a strip, say the subset R × [-1, 1], and are discrete. That is, there are no elements which move points an arbitrarily small distance.
[For example, the additive group of reals R acting on the line by addition is not a discrete group.]
The finite symmetry group of the strip is D2. The elements of this group are: the identity I, a reflection R in the horizontal, a reflection V in a vertical line (through 0 say) and a half turn H (about 0 say).
We have R2 = V2 = H2 = I , RV = VR = H etc.
What else could be in an infinite group?
It could contain a translation T (which we will take to be by a shortest distance in the group) and/or a glide reflection G (which we can take to be G = TR = RT).
We then get the following possible groups generated by some of these elements. In each case we give a pattern which is mapped to itself by the group.
(See Exercises 4 Question 3)
| Group generated by: | Pattern left invariant | Group | |
| (i) | T | .... 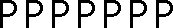 .... .... | C∞ |
| (ii) | G | .... 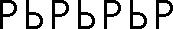 .... .... | C∞ |
| (iii) | T, R | .... 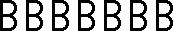 .... .... | C∞ × D1 |
| (iv) | T, V | ....  .... .... | D∞ |
| (v) | T, H | ....  .... .... | D∞ |
| (vi) | G, V | ....  .... .... | D∞ |
| (vii) | everything | .... 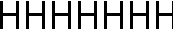 .... .... | D∞ × D1 |
| Previous page (Symmetry groups of plane figures) | Contents | Next page (Symmetry groups of Platonic solids) |