(Just map [u]
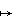 [T(u)] where u are homogeneous coordinates.)
[T(u)] where u are homogeneous coordinates.)However, maps of the form λI for λ a non-zero scalar act as the identity on P(V).
| Previous page (Homogeneous coordinates) | Contents | Next page (More projective groups) |
Any invertible map T from a vector space V to itself leads to a bijection of the associated projective space P(V).
(Just map [u] 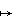 [T(u)] where u are homogeneous coordinates.)
[T(u)] where u are homogeneous coordinates.)
However, maps of the form λI for λ a non-zero scalar act as the identity on P(V).
Definition
If F is any field, the quotient group GL(n, F)/{λI | λ ∈ F - {0} } is called the projective group and is written PGL(n, F).
The elements of this group are called projective transformations or projectivities. (The name projection is used for something different.)
We will now look at some examples
The easiest (and most important) case is PGL(2, R).
This is the group which acts on P(R2) = RP1: the real projective line.
In Klein's formulation it is the group of 1-dimensional real projective geometry.
Let 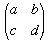 represent an element of PGL(2, R). Note that
represent an element of PGL(2, R). Note that 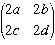 etc. will represent the same element.
etc. will represent the same element.
This acts on an ordinary point with homogeneous coordinates [x, 1] to give [ax + b , cx + d] or equivalently [(ax + b)/(cx + d), 1] provided that cx + d ≠ 0.
That is, the matrix corresponds to the rational map x 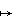 (ax + b)/(cx + d) where we interpret this as ∞ if cx + d = 0. Note that we must have ad - bc ≠ 0 otherwise the map would not be invertible.
(ax + b)/(cx + d) where we interpret this as ∞ if cx + d = 0. Note that we must have ad - bc ≠ 0 otherwise the map would not be invertible.
Looking at what happens to the point at infinity = [1, 0], this maps to a/c (just as we might expect).
Remarks
The following trick makes studying projectivities easier.
Definition
The standard reference points on RP1 are ∞, 0 ,1 (that is [1, 0], [0, 1] and [1, 1]).
Theorem
There is a unique projective transformation taking any three distinct points to ∞, 0 ,1.
Proof
The map is x 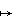 (x - b)/(x - a) . (c - a)/(c - b).
(x - b)/(x - a) . (c - a)/(c - b).

Corollary
There is a unique projective transformation taking any three distinct points to any other three distinct points.
Proof
If the map θ maps a, b, c to ∞, 0 ,1 and φ maps a', b', c' to ∞, 0 ,1 then the map φ-1θ does what is needed.

Projective maps do not preserve lengths or ratios or even intermediacy. However they do preserve something called cross-ratio.
Definition
Let a, b, c, d be four points of RP1. Let θ be the map taking a, b, c to ∞, 0, 1. Then the cross-ratio (a , b ; c , d) is θ(d).
From the above this is (a , b ; c , d) = (d - b)/(d - a) . (c - a)/(c - b).
Remarks

Proof
If f is a projective map and θ takes a, b, c to ∞, 0, 1 then θ  f -1 takes f(a), f(b), f(c) to ∞, 0, 1. The cross ratio (a , b ; c , d) is θ(d) and this is the same as θ
f -1 takes f(a), f(b), f(c) to ∞, 0, 1. The cross ratio (a , b ; c , d) is θ(d) and this is the same as θ  f -1(f(d)) and the result follows.
f -1(f(d)) and the result follows.

| Previous page (Homogeneous coordinates) | Contents | Next page (More projective groups) |