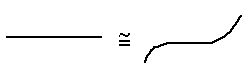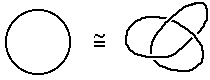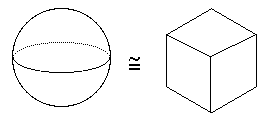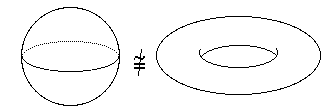Introduction to topology

Topology is (roughly) the study of properties invariant under "continuous transformation".

More formally:
Let S, T be sets with a structure for which the idea of continuity makes sense. e.g. Subsets of Rⁿ, metric spaces, ...
Then a bijection f from S to T is called a homeomorphism or topological isomorphism if both f and f^-1 are continuous. We then write S T.
In Klein's formulation, the set of all such maps from a space to itself is a group and topology is the associated geometry.

Examples

1. A line and a curve are homeomorphic:

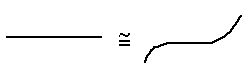
2. A circle S¹ and a knot K (subsets of R³) are homeomorphic -- even though one cannot be deformed into the other (in R³).
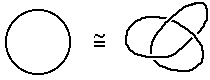
   Strangely, R³ - S¹ and R³ - K are not homeomorphic.

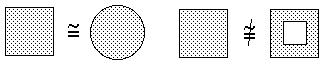
3. A closed (including its boundary) disc and closed unit square are homeomorphic.

4. A sphere (the surface) and the surface of a cube are homeomorphic.
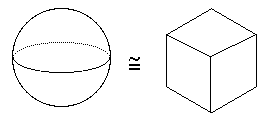
   "Proof"
   Put the cube at the centre of the sphere and project from the centre.

5. A sphere and a torus are not homeomorphic.
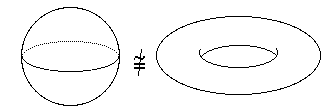
   "Proof"
   Removing a circle from a sphere always splits it into two parts -- not so for the torus.

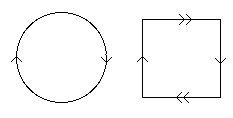
6. A cylinder S1 × I and the space made by gluing a strip after a full turn are homeomorphic -- even though (cf example 2) one cannot be deformed into the other.

7. The Möbius band is a "space" made by gluing together the ends of a strip after a half turn.

8. The Real projective plane RP¹ = {the set of lines through 0 in R³}
   If we take an "upper hemisphere", this meets each line in a unique point which we may take as the representative of that point of the projective plane -- except for opposite points on the equator which have to be "identified".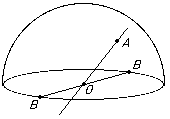
   Hence the Real Projective Plane can be regarded as a disc which opposite points on its boundary identified.