Course MT4521 Geometry and topology
Symmetry groups of plane figures
We now consider some interesting subgroups of I(R2).
Following the spirit of Klein's Erlangen program we look for properties preserved by these subgroups. In particular we look for subsets X of S = R2 which are mapped to themselves by all the elements of these subgroups.
The subgroup is then the symmetry group of the subset X.
Examples
- Subgroups generated by a rotation
Rotation by 2π/n generates a subgroup isomorphic to Cn the cyclic group of order n.
Note that Cn is the symmetry group of many figures in R2.
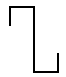 has symmetry group C2 ,
has symmetry group C2 , 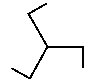 has symmetry group C3 ,
has symmetry group C3 , 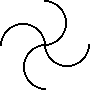 has symmetry group C4 , etc.
has symmetry group C4 , etc.
- Dihedral groups
The Dihedral group Dn is the group of symmetries of a regular n-gon. It has order 2n.
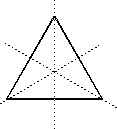 The group D3 consists of rotations by 0, 2π/3 and 4π/3 and three reflections.
The group D3 consists of rotations by 0, 2π/3 and 4π/3 and three reflections.
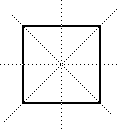 The group D4 consists of four rotations by multiples of π/2 and four reflections -- two through lines joining vertices and two through lines joining mid-points of sides.
The group D4 consists of four rotations by multiples of π/2 and four reflections -- two through lines joining vertices and two through lines joining mid-points of sides.
One may show that in terms of generators and relations, Dn= < a, b | an = b2 = 1, bab = a -1 >.
For n ≥ 3 it is a non-abelian group. The group D3  S3 (the symmetric group on three symbols).
S3 (the symmetric group on three symbols).
The group D2 is the symmetry group of a "regular 2-gon"  and consists of the identity, rotation by π and reflections in two perpendicular lines.
and consists of the identity, rotation by π and reflections in two perpendicular lines.
It is called the Klein 4-group. It is sometimes written V and is isomorphic to C2× C2 .
The group D1 is the group consisting of the identity and reflection in a line: 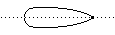
Note that as a group this is a cycle group of order 2 but that it is not the same subgroup as any of the subgroups C2 of (1) above which are generated by rotations.
Remark
Note that for each point p of R2 there is a subgroup in I(R2) isomorphic to Cn and many subgroups isomorphic to Dn (corresponding to the different directions that one could choose for the mirrors through p).
Theorem (Leonardo da Vinci about 1500)
The above examples Cn and Dn for some positive integer n are the only finite subgroups of I(R2).
Proof
Let G be a finite subgroup of I(R2). Then G cannot contain a translation or glide reflection since these elements have infinite orders.
It cannot contain rotations about two distinct points otherwise (See Exercises 3 Question 6) it would contain a translation.
If it contains a rotation about a point a, it cannot contain a reflection in any line not through a (See Exercises 3 Question 2).
It can only contain reflections in lines through a common point (Otherwise one could combine two of them to get a rotation about one of the meeting points and a reflection through a different point).
Hence there is a point of R2 left fixed by all the elements of G.
Take this point as the origin and then G  O(2).
O(2).
H = G SO(2) contains only rotations and hence contains a smallest (non-zero) rotation. All the others must be multiples of this one (otherwise one could combine them to get a rotation whose magnitude was their hcf) and so we have H  Cn .
Cn .
If G ≠ H then H has two cosets in G (since SO(2) has two cosets in O(2)) and the reflections form a coset rH and it is easy to verify that if h is a generator of H then rhr = h -1 .
That is, G  Dn .
Dn .

Remark
In fact (See Exercises 4 Question 1) any two copies G1 and G2 of the groups Cn of rotations are conjugate in I(R2). That is, for some element g ∈ I(R2) we have g G1 g-1 = G2. Similarly any two copies of the same dihedral group are also conjugate. Thus the classification of the finite subgroups of I(R2) is up to conjugacy in I(R2).
JOC February 2010
 has symmetry group C2 ,
has symmetry group C2 , 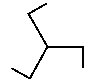 has symmetry group C3 ,
has symmetry group C3 , 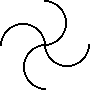 has symmetry group C4 , etc.
has symmetry group C4 , etc.
 The group D3 consists of rotations by 0, 2π/3 and 4π/3 and three reflections.
The group D3 consists of rotations by 0, 2π/3 and 4π/3 and three reflections.
 The group D4 consists of four rotations by multiples of π/2 and four reflections -- two through lines joining vertices and two through lines joining mid-points of sides.
The group D4 consists of four rotations by multiples of π/2 and four reflections -- two through lines joining vertices and two through lines joining mid-points of sides.
 S3 (the symmetric group on three symbols).
S3 (the symmetric group on three symbols).
 and consists of the identity, rotation by π and reflections in two perpendicular lines.
and consists of the identity, rotation by π and reflections in two perpendicular lines.
 O(2).
O(2).