Metric and Topological Spaces
Previous page
(Definition and examples of metric spaces) 		Contents Next page
(Continuity in metric spaces)

Convergence in metric spaces

The definitions given earlier for R generalise very naturally.

Definition
The sequence (xn) in a metric space is convergent to x belongs X if:

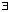Given epsilon > 0 thereexists N belongs N such that n > N implies d(xn, x) < epsilon.

One may rephrase this as
(xn) rarrow x in the metric space X if the real sequence (d(xn , x)) rarrow 0 in R.

Examples

  1. The plane R2 with various metrics:
    It turns out that for the metrics d1, d2 , dinfinity if a sequence is convergent in one metric, it is convergent in the others.
    In fact for these metrics a sequence ((xi , yi)) in R2 converges to (x, y) if and only if (xi) rarrow x and (yi) rarrow y in R. That is, convergence is componentwise.
    However, you should note that for any set with the discrete metric a sequence is convergent if and only if it is eventually constant.
    So whether the sequence converges or not may depend on what metric you are using.

    This becomes even more important in:

  2. C[0, 1] with various metrics.
    Take the sequence (fn) with fn the function whose graph is:
    Then d1(f1 , 0 ) = 1/n (where 0 is the zero-function) and so this sequence converges to the zero-function in d1.
    However, in dinfinity we have dinfinity(fn , 0) = 1 for all n and so this sequence does not converge to the zero-function in the metric dinfinity. In fact it does not converge took any function.

    We will look at C[0, 1] with the dinfinity-metric in more detail later.

Previous page
(Definition and examples of metric spaces) 		Contents Next page
(Continuity in metric spaces)
JOC February 2004