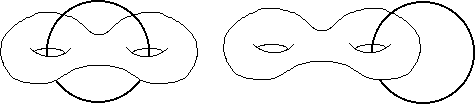Exercises 1

1. Consider the upper-case letters of the alphabet { A, B, C, D, ... , Z } as being made up of (infinitely thin) lines. Classify them up to topological equivalence.
   If we treat them as being made of lines of finite thickness (so that they are two-dimensional sets) how does the classification change?
   If we treat them as being carved out of (say) wood (so that they are three-dimensional sets) does the classification change again?

   Solution to question 1

2. Recall the definitions:
   A closed interval is the set [a, b] = {x R | a x b }
   An open interval is the set (a, b) = {x R | a < x < b } and there are also open intervals
   (a, ) = {x R | a < x }, (-, b) = {x R | x < b } and (-, ) = R.
   A half-open interval is the set [a, b) = {x R | a x < b } or (a, b] = {x R | a < x b } and there are also half-open intervals [a, ) and (-, b] defined similarly.
   Draw the graphs of continuous maps which show that any two closed intervals are homeomorphic (topologically equivalent).
   Prove that any two finite open intervals are homeomorphic.
   Prove that the open interval (-p/2, p/2) is homeomorphic to the real line R.
   [Hint: Consider the map f(x) = tan(x).]
   Prove that any two open intervals are homeomorphic.
   Prove that any two half-open intervals are homeomorphic.

   Solution to question 2

3. Draw the graph of a continuous function from the open interval (0, 1) onto the closed interval [0, 1].
   Can you find a continuous function from the interval [0, 1] onto the interval (0, 1) ?

   Solution to question 3

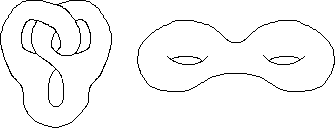4. Show that the two embeddings of (the surface of the) two-holed torus shown on the right can be deformed into one another in R³.
   [Hint: Think of the two-holed torus as being made from a sphere by attaching two "tubes".]
   
   
   Show how the two views below of a ring and a two-holed torus can be deformed into one another in R³.

   

   Solution to question 4