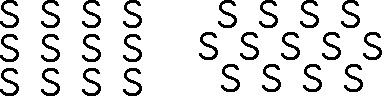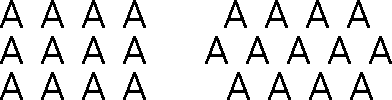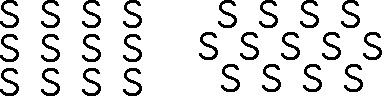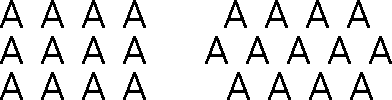Course MT3818 Topics in Geometry
Classification of crystallographic groups
Our aim is to classify all the discrete subgroups of I(R2). When we classified subgroups of I(R2) and I(R3) earlier, we classified them up to conjugacy inside the groups of all symmetries. This time, however, the appropriate notion of equivalence is a bit different.
Definition
We will call two plane symmetry groups G and H equivalent if there is an isomorphism from G to H which maps the lattice of G to the lattice of H.
Remarks
- The lattices of two such equivalent groups will be the same and hence their point groups will also agree. The action of the point groups on the lattices will also be mapped across by the isomorphism as will shift vectors of elements of the point groups.
- This notion is coarser than being conjugate subgroups. For example, the symmetry groups of two different sized or shaped rectangular lattices will be different up to conjugacy, but will be the same under this equivalence.
- There are several other ways of defining this equivalence.
Examples
- The groups of the two patterns below are equivalent but are not conjugate subgroups of I(R2).
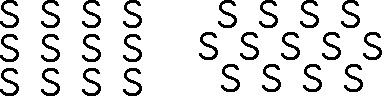
- The groups of the two patterns below are not equivalent.
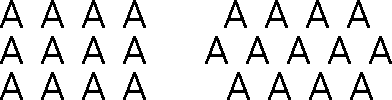
We split up the classification into several stages
JOC March 2003