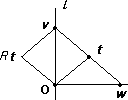
This is the case cm.
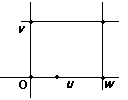
This is the case pm.

This is the case pg.
| Previous page (I: Point group is cyclic) | Contents | Next page (III: Other point groups) |
The group D1 is generated by a reflection. The result for this section is:
Theorem
There are three equivalence classes of symmetry group with point group D1.
Proof
The group D1 is generated by a reflection R in a line l.
Choose a minimal length vector v of the lattice L lying on l. (See the example of shift vectors considered above.)
Then choose a vector w in the lattice perpendicular to L and of minimal length.
Note that for any u ∈ L the vector u - R(u) is perpendicular to l since R(u - R(u)) = -(u - R(u)).
Then there are three possibilities.



 cm |  pm |  pg |
| Previous page (I: Point group is cyclic) | Contents | Next page (III: Other point groups) |