Exercises 8

1. Let f be the element f = (ax + b)/(cx - a) with a, b ∈ R, a² + bc > 0. Find the order of f in the projective group PGL(2, R).
   Prove that f has two fixed points, p, q equidistant from f(∞).
   If x ≠ p, q is a point in RP¹, prove that the cross-ratios ( p , q ; x , f(x) ) = ( p , q ; f(x) , x ) and deduce that these are -1.

   Solution to question 1

2. Show that any three distinct points on a projective line can be mapped to any three distinct points on a second line by positioning the lines suitably in a plane and projecting from a point not on either line.

   Solution to question 2

3. Show that any four points (no three on a line) in RP² can be mapped to any other four such points by a projective transformation.
   [Hint: Show first that one can map [α, 0, 0], [0, β, 0] and [0, 0, γ] to three of the points. Then choose α, β, γ so that [1, 1, 1] is mapped to the fourth point.]

   Solution to question 3

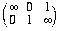4. Write down a formula for a projective transformation which corresponds to the cyclic permutation of the set { ∞, 0, 1 }.
   If a, b, c are any distinct points of RP¹, describe how to find a projective transformation of order 3 which maps a to b and b to c.

   Solution to question 4

5. If a matrix A ∈ GL(2, R) represents an element f of PGL(2, R), prove that the eigenvectors of A correspond to the fixed points of f.
   Show that the matrix 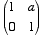with a ≠ 0 represents an element T_a of PGL(2, R) which has ∞ as a unique fixed point and that restricted to the affine part of RP¹ it represents a translation by a.
   Prove that any projective map with a single fixed point is conjugate to a map of the form T_a for some a.

   Solution to question 5

   
   

6. A pencil of lines is a set of lines through a common point.
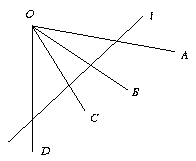
   If a line l meets the pencil OA, OB, OC, OD as shown, show that the cross-ratio of the four points of intersection does not depend on which line l we take.

   Solution to question 6
   
   
   

7. Prove Pappus's theorem (about 320 AD):
   If the vertices of a hexagon lie alternately on two lines, then the meets of opposite sides are collinear.
   [Hint: Project two of the meets to points on the line at infinity.]

   Solution to question 7

8. State the dual of Pappus's theorem.

   Solution to question 8