 and X are closed
and X are closed A
A  B is closed
B is closed I} closed
I} closed 
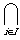 Ai is closed
Ai is closed| Previous page (Definition and examples of topologies) | Contents | Next page (Continuity for topological spaces) |
We can recover some of the things we did for metric spaces earlier.
Definition
A subset A of a topological space X is called closed if X - A is open in X.
Then closed sets satisfy the following properties
 and X are closed
and X are closed A
A  B is closed
B is closed I} closed
I} closed 
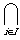 Ai is closed
Ai is closedSo the set of all closed sets is closed [!] under finite unions and arbitrary intersections.
As in the metric space case, we have
Definition
A point x is a limit point of a set A if every open set containing x meets A (in a point 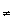 x).
x).
Theorem
A set is closed if and only if it contains all its limit points.
Proof
Imitate the metric space proof.

Definitions
The interior int(A) of a set A is the largest open set  A,
A,
The closure cl(A) of a set A is the smallest closed set containing A.
It is easy to see that int(A) is the union of all the open sets of X contained in A and cl(A) is the intersection of all the closed sets of X containing A.
Some properties
 ) =
) = 
 cl(A) for any subset A
cl(A) for any subset A B) = cl(A)
B) = cl(A)  cl(B) for any subsets A and B
cl(B) for any subsets A and B
To prove K3. note that cl(A)  cl(B) is a closed set which contains A
cl(B) is a closed set which contains A  B and so cl(A)
B and so cl(A)  cl(A
cl(A  B).
B).
Similarly, cl(B)  cl(A
cl(A  B) and so cl(A)
B) and so cl(A)  cl(B)
cl(B)  cl(A
cl(A  B) and the result follows.
B) and the result follows.
To prove K4. we have cl(A)  cl(cl(A)) from K2. Also cl(A) is a closed set which contains cl(A) and hence it contains cl(cl(A)).
cl(cl(A)) from K2. Also cl(A) is a closed set which contains cl(A) and hence it contains cl(cl(A)).

Remark
These four properties are sometimes called the Kuratowski axioms after the Polish mathematician Kazimierz Kuratowski (1896 to 1980) who used them to define a structure equivalent to what we now call a topology.
Examples
 ={
={  , X, {a} } cl({a}) = X and int({b}) =
, X, {a} } cl({a}) = X and int({b}) = 
 = {
= { , R}
, R}  { (x,
{ (x,  ) | x
) | x  R} then cl({0}) = (-
R} then cl({0}) = (- , 0] and int( (0, 1) ) =
, 0] and int( (0, 1) ) = 
| Previous page (Definition and examples of topologies) | Contents | Next page (Continuity for topological spaces) |