Exercises 3

1. If f, g are continuous functions on an interval [a, b], prove that d₁(f, g) (b - a)d(f, g).
   Hence prove that if a sequence (f_n) in C[a, b] converges to a function f in d it also converges to f in d₁.

   Solution to question 1

2. A sequence (x_i) in a metric space (X, d) is called a Cauchy sequence if:
   Given > 0, there exists N N such that m, n > N d(x_m, x_n) < .
   Let (f_n) be the sequence of functions on [-1, 1] defined by
   f_n(x) = 0 for x 0, f_n(x) = nx for 0 < x < ¹/_n and f_n(x) = 1 for x 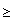¹/_n.
   Sketch the graphs of a typical pair of functions f_m and f_n and hence or otherwise prove that the sequence (f_n) is a Cauchy sequence in C[-1, 1] with the metric d₁.
   Calculate the pointwise limit of the sequence (f_n) and hence prove that the sequence (f_n) is not a convergent sequence in C[-1, 1] under this metric.

   Solution to question 2

3. Let f be a map from a metric space X to a metric space Y. If the metric on X is the discrete metric, prove that f is continuous. If the metric on Y is discrete, is f necessarily continuous ?

   Solution to question 3

4. This question tries to show you why the metric d₂ on a space of continuous functions is an easier one to work with than d₁ or d.
   
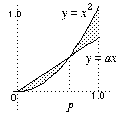   Find the best-fit straight-line through the origin to the function x² in the metric d₁ .
   That is, find the value of a which minimises d₁(x², ax).

   For this you have to minimise the shaded area. Calculate this area by first finding the x-coordinate of the point P in terms of a.
   
   
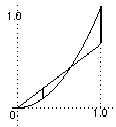   Find the best-fit straight-line through the origin to the function x² in the metric d .
   That is, find the value of a which minimises d(x², ax).
   For this you have to choose a so that the "vertical distance between the graphs" is a minimum. From the picture, you can see that the best you can do is to adjust the value of a so that the two dark lines have equal length.
   
   
   Calculate d₂(x², ax) and then use the usual calculus method for finding the maximal value of a function to find the best-fit straight-line through the origin to the function x² in the metric d₂ .

   Solution to question 4