Limits of functions

Our definition of continuity allows us to talk about the limit of a function.

Definition

If the sequence (f (x_n) converges to the same limit for any sequence with (x_n)→ p with x_n≠ p we call this limit f (p).

We can then rephrase the definition of continuity given above as:

A function f is continuous at p if f (x) exists and is equal to f (p).

Variations of the above definition are:

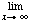f (p). [Take (x_n) to be any unbounded monotonic increasing sequence.]

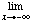f (p). [Take (x_n) to be any unbounded monotonic decreasing sequence.]

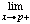f (p). [Take (x_n) to be any sequence converging to p with x_n> p.]

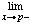f (p). [Take (x_n) to be any sequence converging to p with x_n< p.]

1. The condition x_n≠ p in the above definition is to allow for the possibility that f (x) is not defined at the point x = p. This is, for example, the case in the definition of the derivative of a function.
   
2. In terms of quantifiers, we may define a function to be continuous if:
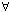   (∀p ∈ R)(ε > 0)(∀x ∈ R)(∃δ > 0)(|x - p| < δ ⇒ |f (x) - f (p) < ε)
   Note that the value of δ that we need to find is allowed to depend on x as well as on ε.
   It is common for beginners to mis-state the definition as:
   (∀p ∈ R)(∀ε > 0)(∃δ > 0)(∀x ∈ R)(|x - p| < δ ⇒ |f (x) - f (p) < ε)
   In this case the same value of δ would have to work for all ε.